|
Lesson 12 - Neutron sources
Chapter 4 is concerned with the description of the physical mechanisms
that give rise to neutron, gamma ray, or x-ray sources. The chapter
forms a excellent reference for all sorts of useful information, but we
are going to be more selective with the material we concentrate on.
Source description is the first step of any shielding analysis.
In many cases, the particle sources will be known from:
-
The problem specification (especially for "made up" problems for method
verification studies or from standardized conservative sources specified
by some industry standard); or
-
A previous analysis (especially for reactor shielding studies).
The other cases is the situation where material properties
are specified and the analyst has to deduce the source from this known
material. This is the situation that we will be concentrating
on.
Basically, we will be looking at the MAJOR sources of particles that
I think you should be aware of, with an eye toward sensitizing you to look
for sources that are likely to "sneak up" on you if you are not careful.
(Our greatest danger is to underestimate a source because we overlook a
complete category.)
The book lists 5 neutron source categories:
-
Fission sources
-
Photoneutron sources
-
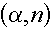sources
-
Activation neutrons
-
Fusion neutrons
From the reading, you should be able to describe the physical mechanism
for each of these (as I had you do in the Reading Assignment) and work
very simple problems that require basic skills you already have (e.g.,
atom density determinations for nuclides, time-dependent buildup and decay,
reaction rate determination from fluxes and cross sections).
In addition, we will be covering more detail about two of these, which
are the mechanisms of spontaneous fission and sources due to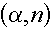
reactions. In addition, as the last section of
this lesson, we will briefly explore the idea of multigroup energy representation.
Spontaneous Fission Sources
The first of the neutron that we will concentrate on is spontaneous fission,
which is a version of fission that occurs without the benefit of an inducing
neutron being absorbed. Several notes:
-
Since there is no incoming neutron, the reaction doesn't have the advantage
of the neutron's kinetic and (especially) binding energy to speed up the
reaction. Therefore, the reaction time is slow, with half-lives
in years instead of nanoseconds.
-
Because there is no incoming neutron, the underlying nucleus must "supply"
it. This is a strange way of saying that the predominant spontaneous
fission nuclides have a mass one greater than the predominant induced
fission nuclides. For example, the primary fissile plutonium isotopes
are the odd isotopes, Pu-235, -237, -239, -241, and -243; the corresponding
primary spontaneous fission nuclides are the even isotopes, Pu-236,-238,
-240, -242, and Pu-244.
-
This is a single-nuclide reaction, so it is independent of the chemical
or physical form of the underlying material, but depends only on its mass.
When considering any of these sources, we are concerned with two factors:
the yield (number of particles produced) and the spectrum
(energy distribution of the particles produced).
For spontaneous fission, the yield depends only on the underlying mass;
the most convenient form of data for this is usually something like the
last column of Table 4.2. You should be able to determine spontaneous
fission yields with data from this table.
Example: What is the neutron source rate from a 1 microgram point
source of Cf-252?
Answer: From Table 4.2, the spontaneous fission yield of 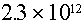 neutrons/sec/gram.
Therefore the source rate is:
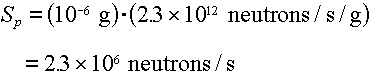
For the spectrum, we generally use the Watt spectrum form given by Equations
4.1 and 4.2 (with data from Table 4.3). Note from Figure 4.1 that
all nuclides have a similar shape, differing primarily in the high-energy
"tail". (Note that the plotted spectra differ by about a factor of
10 at 15 MeV in Figure 4.1.) This difference can be more significant
than the logarithmic y-axis of this figure implies, since it is these high
energy particles that are most likely to penetrate shielding.
Notice, though, the mixed nature of Figure 4.1 . For all isotopes
except Cf-252, the curve is for induced fission; therefore, to use
the figure for spontaneous fission, one has to recognize that the
spontaneously fissioning nuclide is the nuclide we have AFTER the inducing
neutron has been absorbed. For example, the curve for induced Pu-239
fission corresponds to spontaneous Pu-240 fission (since, of course, for
a neutron causing a fission in Pu-239, the nuclide that breaks apart is
the compound nucleus Pu-240).
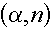Sources
The second neutron source mechanism that we will concentrate on is the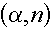
reaction. The physical mechanism of this is that an energetic
alpha particle (produced by an emitter nuclide) breaks through the
Coulomb barrier of the nucleus of a second nuclide (called the
converter
nuclide) and is absorbed. Subsequently, the compound nucleus emits
a neutron.
Several notes:
-
The partnership between the emitter and the converter is a Mutt-and-Jeff
relationship. Most alpha emitters tend to be very heavy nuclides.
(Below a certain point in the periodic chart, radioactive nuclides tend
to decay with beta emission.). In contrast, most converter nuclides
have to be very light nuclides, since the Coulomb barrier of a nucleus
rises as the charge of the nucleus increases. (See Table 4.6.)
-
The Coulomb barrier is usually the minimum energy that the alpha particle
must have to cause an
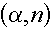reaction in the converter, but sometimes the threshold energy is the minimum
energy. (In the Table 4.6 data, this only happens for the two Li
isotopes.)
-
Because of this threshold energy, the alpha particles are only "at risk"
of inducing an
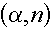reaction from their birth energy to the threshold energy. Of course,
if the alpha particle's energy goes below the threshold energies of all
isotopes in the material, it cannot enter any nucleus and is destined to
slow down, collect a couple of electrons, and go through life as a helium
atom.
-
Also because of the threshold energy (and the fact that alpha particle
ranges go up faster than E),
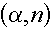yields go up faster than E as well. Equation 4.7 gives us the optimum
yield in Be as a function of alpha energy: As you can see, the yield
goes up even faster than E squared.
-
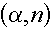yields are LOW. Note that Table 4.7 gives the number of neutrons
per MILLION alpha particles.
Let's discuss "optimum yield". As mentioned in the book, the highest
yield that can come from a given energy alpha in a given converter material
will occur when the alpha slows down in a medium that is "pure" converter.
For example, the optimum yield of 0.000110 neutrons/alpha for Cm-242/Be
corresponds to the characteristic Cm-242 alphas being released into a material
that is 100% Be.
Why does this matter? Well, alpha particles have a limited range.
So, if they are going to interact with Be to produce the neutrons, the
yield is going to be directly proportional to the number of Be nuclei that
the alpha comes into contact with before it slows down below the Be threshold
of 2.6 MeV.
The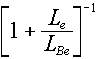 
part of the book's Equation 4.6 is there to take care of this factor, where
the L factors are stopping powers for the emitter and the converter (Be,
in this case). Rather than get caught up in complicated determinations
of these stopping powers -- which was covered in a part of Chapter 3 that
we didn't study (note also that the description of Equation 4.6 in the
text refers you to "Section 3.9", which doesn't exist), we are going to
use the simplified idea that stopping power is approximately proportional
to electron density:
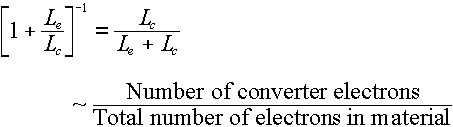
Example: What would the approximate neutron yield (in neutrons/alpha)
be for  ?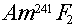
Answer: The optimum yield for Am-241/F is given in Table
4.7 as 4.1 neutrons/million alphas, or 0.0000041= 4.1E-6 neutrons/alpha.
Since this is not a pure F material, we reduce this by the ratio of F electrons
to total electrons:
Since F is element number 9, number of fluorine electrons per molecule
is 2 * 9 = 18.
Since Am is element number 95, the total number of electrons in a molecule
is 18 + 95 = 113.
Therefore, the approximate mixture neutron yield would be 4.1E-6*18/113
= 6.5E-7.
Again, once we have the neutron yield (and from a given problem's material
composition, we can easily convert this to a neutron source), we have to
consider the energy distribution of the neutrons that are produced (i.e.,
the emitted neutron spectrum). The continuous energy
spectra for various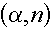
emitter-converter combinations are given in Figure 4.3 in the text.
Multigroup energy representation
Most industry standard computer codes (MCNP being the primary exception)
do not represent energy-dependent data as continuous functions (or distributions),
but instead approximate the continuous data as histograms (i.e.,
stair-step functions) which are constant over sub-divisions of the energy
range. These energy subdivisions are refereed to as "energy groups"
and are, by historical convention, numbered sequentially from high to low
energies. For example, if we divide the neutron energy range logarithmically
(which is standard for neutron groups) from 1 keV to 20 MeV with 3 groups
per decade, we would get the following structure:
|
Group number, g
|
Upper limit of group,  |
| 1 |
(
= ) 20. MeV |
| 2 |
(
= )10. |
| 3 |
5. |
| 4 |
2. |
| 5 |
1. |
| 6 |
0.5 |
| 7 |
0.2 |
| 8 |
0.1 |
| 9 |
0.05 |
| 10 |
0.02 |
| 11 |
0.01 |
| 12 |
0.005 |
| 13 |
0.002 |
| 14 |
0.001 = 1 keV |
Note that the highest energy limit (i.e., the top of group 1) is called .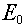
Also, this is just an example. Usually neutron energy group
structures go down to thermal range, bottoming out at about 0.001 eV (=0.000000001
MeV).
Multigroup representations of distributions
For a distribution,  ,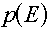
(which, you will remember would have "per unit energy" units), the corresponding
group value is found by a simple integration over the energy range of the
group:
The best examples of when you would do this are the energy spectra (e.g.,
the Watt fission spectrum) described in this lesson. The resulting
group value corresponds to the fraction of produced particles that
would be emitted in the given group.
Note: Be sure that the sum of your group values is 1.0.
If not, you will need to normalize the values by dividing each group value
by the sum of all group values.
For example, if we normalize the Watt spectrum representation of the Cf-252
fission spectrum (given by Equation 4.2 with Table 4.3 data inserted):
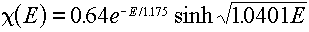
we would get the following values (in the above energy group structure):
|
Group number, g
|
Upper limit of group, |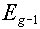
 |
| 1 |
20. MeV |
0.00231 |
| 2 |
10. |
0.06579 |
| 3 |
5. |
0.35378 |
| 4 |
2. |
0.28434 |
| 5 |
1. |
0.16783 |
| 6 |
0.5 |
0.09007 |
| 7 |
0.2 |
0.02269 |
| 8 |
0.1 |
0.00845 |
| 9 |
0.05 |
0.00355 |
| 10 |
0.02 |
0.00079 |
| 11 |
0.01 |
0.00028 |
| 12 |
0.005 |
0.00011 |
| 13 |
0.002 |
.00003 |
| 14 |
0.001 = 1 keV |
.00001 |
NOTE: The integrations were done using an S32 Gauss-Legendre
quadrature integration. The FORTRAN coding for this is given here,
in case you are interested.
You can "eyeball" a spectrum from a spectrum plot by estimating the average
value over the group range and multiplying by the group width.
Example: Verify that the above value for group 3 is reasonable
using Figure 4.1 in the text.
Answer: Group 3 goes from 2 MeV to 5 MeV. The value
of the function falls over the group from about 0.2 (or so) to 0.03.
If we take the average of these as an average value and multiply by the
group width, we get 0.115*3 = 0.345 as our estimate. This compares
okay with the 0.354 value in the table.
Multigroup representations of functions
Although in this lesson we are only interested in distributions, for completeness
it should be mentioned that for functions of energy (e.g., cross sections)
the group value corresponds to the average value of the function over the
energy range, so we have to divide by the energy width. For example,
for a function  ,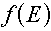
the group value would be given by the equation:
|
NE406 Radiation Protection and Shielding